image
imagewidth (px) 54
2.52k
| pid
stringlengths 2
4
| question
stringlengths 41
810
| options
sequencelengths 0
5
| answer
stringclasses 57
values | solution
stringlengths 0
2.24k
⌀ | subject
stringclasses 1
value | task
stringclasses 1
value | category
stringclasses 1
value | source
stringclasses 1
value | type
stringclasses 2
values | context
stringclasses 1
value |
|---|---|---|---|---|---|---|---|---|---|---|---|
1005 | Carl composed the figure shown on the left side of the drawing from the smaller three-square and four-square figures shown on the right side. The smaller figures can be turned around, but not turned over. What is the smallest number of three-square figures needed for that?
<image1> | [] | 2 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1009 | An equilateral triangle $A C D$ is rotated counterclockwise around point $A$. At what angle has it been rotated unen it covers equilateral triangle $A B C$ for the first time?
<image1> | [
"$60^{\\circ}$",
"$120^{\\circ}$",
"$180^{\\circ}$",
"$240^{\\circ}$",
"$300^{\\circ}$"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1019 | Two rectangles $A B C D$ and $D B E F$ are shown in the figure. What is the area (in $\mathrm{cm}^{2}$ ) of the rectangle $D B E F$?
<image1> | [] | 12 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1029 | The first row shows 11 cards, each with two letters. The second row shows rearangement of the cards. Which of the following could appear on the bottom line of the second row?
<image1> | [
"ANJAMKILIOR",
"RLIIMKOJNAA",
"JANAMKILIRO",
"RAONJMILIKA",
"ANMAIKOLIRJ"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
103 | Ana has the cards shown on the left. She chooses several of them to assemble the tower shown on the right. Which cards did she not use?
<image1>
<image2>
<image3> | [
"A",
"B",
"C",
"D",
"E"
] | C | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1032 | How many little squares at least do we have to shade in the picture on the right in order that it have an axis of symmetry?
<image1> | [] | 3 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1035 | By drawing 9 segments (5 horizontal and 4 vertical) one can make a table of 12 cells. How many cells can you get maximally if you draw 15 segments?
<image1> | [] | 42 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1038 | In the picture there is a square tile with two fourths of a circle. The radius of every fourth is half the side of the tile and its length equals $5 \mathrm{dm}$. We form a large square from 16 such tiles and try to get the longest unbroken curve consisting of the fourths. How long can this continuous curve be at most?
<image1> | [
"$75 \\mathrm{dm}$",
"$100 \\mathrm{dm}$",
"$105 \\mathrm{dm}$",
"$110 \\mathrm{dm}$",
"$80 \\mathrm{dm}$"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1042 | How many squares can be drawn by joining the dots with line segments?
<image1> | [] | 4 | null | Math | 2D Transformation | MathVision | Open-ended | |||
106 | Which of the tiles below is NOT part of the wall next door?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1060 | How many lines of symmetry does this figure have?
<image1> | [] | 2 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1062 | Martina draws the six cornerpoints of a regular hexagon and then connects some of them to obtain a geometric figure. Which of the following figures cannot be generated?
<image1> | [
"trapezium",
"right angled triangle",
"square",
"kite",
"obtuse triangle"
] | C | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1080 | The figure on the left consists of two rectangles. Two side lengths are marked: 11 and 13. The figure is cut into three parts along the two lines drawn inside. These can be put together to make the triangle shown on the right. How long is the side marked $\mathrm{x}$?
<image1> | [] | 37 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1084 | One vertex of the triangle on the left is connected to one vertex of the triangle on the right using a straight line so that no connecting line segment dissects either of the two triangles into two parts. In how many ways is this possible?
<image1> | [] | 4 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1093 | A piece of string is folded as shown in the diagram by folding it in the middle, then folding it in the middle again und finally folding it in the middle once more. Then this folded piece of string is cut so that several pieces emerge. Amongst the resulting pieces there are some with length $4 \mathrm{~m}$ and some with length $9 \mathrm{~m}$. Which of the following lengths cannot be the total length of the original piece of string?
<image1> | [
"$52 \\mathrm{~m}$",
"$68 \\mathrm{~m}$",
"$72 \\mathrm{~m}$",
"$88 \\mathrm{~m}$",
"All answers are possible."
] | C | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1096 | Melanie has a square piece of paper with a $4 \times 4$ grid drawn on it. She cuts along the gridlines and cuts several shapes out which all look either the same as the one pictured, or the same as its mirror image. How many squares are left over if she cuts out as many shapes as possible?
<image1> | [] | 4 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1099 | In the $8 \times 6$ grid pictured, there are 24 squares that have not been cut by either of the two diagonals. Now we draw the two diagonals on a $10 \times 6$ grid. How many squares in this grid will not be cut by either of the two diagonals?
<image1> | [] | 32 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1119 | The word KANGAROO is written on the top of my umbrella. Which of the following pictures shows my umbrella?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
112 | Turning a card around on the top side, we see the photo of the kangaroo. Instead, if we turn the card around on the right side, what will appear?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1127 | A square with area 30 is split into two by its diagonal and then Split into triangles as shown in the diagram. Some of the areas of the triangles are given in the diagram. Which of the line segments $a, b, c, d, e$ of the diagonal is the longest?
<image1> | [
"a",
"b",
"c",
"d",
"e"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1133 | A card has a diagram printed on one side and the other side is plain white. The card is first flipped over downwards and then to the right (see diagram). Which picture is obtained?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | B | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1150 | The black and the dashed line together form seven equilateral triangles. The dashed line is $20 \mathrm{~cm}$ long. How long is the black line?
<image1> | [
"$25 \\mathrm{~cm}$",
"$30 \\mathrm{~cm}$",
"$35 \\mathrm{~cm}$",
"$40 \\mathrm{~cm}$",
"$45 \\mathrm{~cm}$"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1159 | If the letters of the Word MAMA are written underneath each other then the word has a vertical axis of symmetry. For which of these words does that also hold true?
<image1> | [
"ADAM",
"BAUM",
"BOOT",
"LOGO",
"TOTO"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1173 | Lisas aviation club designs a flag with a flying "dove" on a $4 \times 6$-grid. The area of the "dove" is $192 \mathrm{~cm}^{2}$. The perimeter of the "dove" is made up of straight lines and circular arcs. What measurements does the flag have?
<image1> | [
"$6 \\mathrm{~cm} \\times 4 \\mathrm{~cm}$",
"$12 \\mathrm{~cm} \\times 8 \\mathrm{~cm}$",
"$20 \\mathrm{~cm} \\times 12 \\mathrm{~cm}$",
"$24 \\mathrm{~cm} \\times 16 \\mathrm{~cm}$",
"$30 \\mathrm{~cm} \\times 20 \\mathrm{~cm}$"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1184 | A $4 \times 4$ square is made up of the two pieces shown. Which of the following $4 \times 4$ squares cannot be made this way?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
119 | A kangaroo laid out 3 sticks like this to make a shape. It is not allowed to break or to bend the sticks. Which shape could the kangaroo make?
<image1> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1204 | Zilda took a square sheet of paper of side 1 and made two folds taking two consecutive sides of the sheet to a diagonal of it, as shown in the picture, obtaining a quadrilateral (highlighted outline). What is the area of this quadrilateral?
<image1> | [
"$\\frac{7}{10}$",
"$2-\\sqrt{2}$",
"$\\frac{3}{5}$",
"$\\sqrt{2}-1$",
"$\\frac{\\sqrt{2}}{2}$"
] | B | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1206 | Which of the following symbols for signs of the Zodiac has an axis of symmetry?
<image1> | [
"A",
"B",
"C",
"D",
"E"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1208 | When the 5 pieces <image1> are fitted together correctly, the result is a rectangle with a calculation written on it. What is the answer to this calculation? | [] | -100 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1212 | The area of the large square is $16 \mathrm{~cm}^{2}$ and the area of each small square is $1 \mathrm{~cm}^{2}$. What is the total area of the central flower in $\mathrm{cm}^{2}$?
<image1> | [] | 4 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1232 | Gardener Toni plants tulips <image1> and sunflowers <image2> in a square flowerbed with side length $12 \mathrm{~m}$, as shown in the diagram. How big is the entire area where sunflowers are planted?
<image3> | [
"$36 \\mathrm{~m}^{2}$",
"$40 \\mathrm{~m}^{2}$",
"$44 \\mathrm{~m}^{2}$",
"$46 \\mathrm{~m}^{2}$",
"$48 \\mathrm{~m}^{2}$"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1239 | The diagram shows a grid made of vertical and horizontal lines. Which part was cut from the grid? <image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1241 | A dark disc with two holes is placed on top of a dial of a watch as shown. The dark disc is now rotated so that the number 8 can be seen through one of the holes. Which of the numbers could one see through the other hole now? <image1> | [
"4 and 12",
"1 and 5",
"1 and 4",
"7 and 11",
"5 and 12"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1242 | Kristina has a piece of see-through foil on which some points and lines are drawn. She folds the foil along the dotted line. <image1> What can she see now? | [
"$2\\vdots6\\vdots9$",
"$2\\vdots6\\vdots6$",
"$5\\vdots6\\vdots6$",
"$2\\vdots8\\vdots6$",
"$5\\vdots8\\vdots9$"
] | C | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1243 | A $4\times 6$ grid should be cut along the black lines into several identical shapes. No piece is to be left over. Into which of the following shapes is it not possible to cut this grid in this way? <image1> <image2> | [
"A",
"B",
"C",
"D",
"E"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1262 | In the picture, three strips of the same horizontal width $a$ are marked 1,2,3. These strips connect the two parallel lines. Which strip has the biggest area?
<image1> | [
"All three strips have the same area",
"Strip 1",
"Strip 2",
"Strip 3",
"Impossible to answer without knowing $a$"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1270 | A rectangular sheet of paper with measures $6 \times 12$ is folded along its diagonal. The shaded parts sticking out over the edge of the overlapping area are cut off and the sheet is unfolded. Now it has the shape of a rhombus. Find the length of the side of the rhombus.
<image1> | [] | 7.5 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1299 | A square with sides of length 10 is rolled without slipping along a line. The rolling stops when $P$ first returns to the line. What is the length of the curve that $P$ has travelled?
<image1> | [
"$10 \\pi$",
"$5 \\pi+5 \\pi \\sqrt{2}$",
"$10 \\pi+5 \\pi \\sqrt{2}$",
"$5 \\pi+10 \\pi \\sqrt{2}$",
"$10 \\pi+10 \\pi \\sqrt{2}$"
] | C | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
130 | Which figure can be made from the 2 pieces shown on the right?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1304 | An $8 \mathrm{~m}$ long rope is fastened to the corner of the house. A dog is fastened to the rope. Find the perimeter of the area, where the dog can be found.
<image1> | [
"$15 \\pi+16$",
"$15 \\pi+20$",
"$15 \\pi$",
"$15 \\pi+18$",
"$30 \\pi+16$"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1306 | A coin with diameter $1 \mathrm{~cm}$ rolls around the contour outside of a regular hexagon with sides $1 \mathrm{~cm}$ long, as shown. How long is the path traced by the centre of the coin (in $\mathrm{cm}$ )?
<image1> | [
"$6+\\frac{\\pi}{2}$",
"$6+\\pi$",
"$12+\\pi$",
"$6+2 \\pi$",
"$12+2 \\pi$"
] | B | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
132 | Mara built the square by using 4 of the following 5 shapes. Which shape was not used?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1331 | Six points are marked on a square grid as pictured. Which geometric figure cannot be drawn if only the marked points are allowed to be used as cornerpoints of the figure?
<image1> | [
"square",
"parallelogram with different long sides",
"acute triangle",
"obtuse triangle",
"all figures are possible"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1345 | The two bold lines on the right are rotations of each other. Which of the given points could be the centre of this rotation?
<image1> | [
"only $X$",
"$X$ and $Z$",
"$X$ and $T$",
"only $T$",
"$X, Y, Z$ and $T$"
] | C | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1353 | Three big boxes $P, Q$ and $R$ are stored in a warehouse. The upper picture on the right shows their placements from above. The boxes are so heavy that they can only be rotated $90^{\circ}$ around a vertical edge as indicated in the pictures below. Now the boxes should be rotated to stand against the wall in a certain order. Which arrangement is possible?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"All four arrangements are possible."
] | B | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1355 | Which of the shapes to the right has the largest area?
<image1> | [
"A",
"B",
"C",
"D",
"All shapes have the same area."
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1363 | A rectangle $A B C D$ with dimensions $16 \mathrm{~cm}$ by $4 \mathrm{~cm}$ was folded along the line MN so that corner C meets corner A. What is the area of the Pentagon ABNMD'?
<image1> | [
"$17 \\mathrm{~cm}^{2}$",
"$27 \\mathrm{~cm}^{2}$",
"$37 \\mathrm{~cm}^{2}$",
"$47 \\mathrm{~cm}^{2}$",
"$57 \\mathrm{~cm}^{2}$"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1370 | In a drawing we can see a three quarter circle with centre M and an indicated orientation arrow. This three-quarter circle is first turned $90^{\circ}$ anti-clockwise about M and then reflected in the x - axis. Which is the resulting picture?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1371 | Triangle RZT is generated by rotating the equilateral triangle AZC about point Z. Angle $\beta=\angle \mathrm{CZR}=70^{\circ}$. Determine angle $\alpha=\angle \mathrm{CAR}$.
<image1> | [
"$20^{\\circ}$",
"$25^{\\circ}$",
"$30^{\\circ}$",
"$35^{\\circ}$",
"$40^{\\circ}$"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1377 | $a, b$ and $c$ show the lengths of the different of pieces of wire pictured. Which of the following inequalities is correct?
<image1> | [
"$a<b<c$",
"$a<c<b$",
"$b<a<c$",
"$b<c<a$",
"$c<b<a$"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1388 | Mr. Hide wants to find a treasure that he buried years before. He can only remember that he buried the treasure at least $5 \mathrm{~m}$ away from the hedge and no more than $5 \mathrm{~m}$ away from the old pear tree. Which picture shows best the area where Mr. Hide has to look for the treasure?
<image1> | [
"A",
"B",
"C",
"D",
"E"
] | B | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
139 | Anna cuts the picture of a mushroom in two halves.
<image1>
She then arranges the two pieces together to form a new picture. What could this new picture look like?
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1392 | Four objects $a, b, c, d$ are placed on a double balance as shown. Then two of the objects are exchanged, which results in the change of position of the balance as shown. Which two objects were exchanged?
<image1> | [
"$a$ and $b$",
"$b$ and $d$",
"$b$ and $c$",
"$a$ and $d$",
"$a$ and $c$"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1403 | Peter writes the word KANGAROO on a see-through piece of glass, as seen on the right. What can he see when he first flips over the glass onto its back along the right-hand side edge and then turns it about $180^{\circ}$ while it is lying on the table?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1404 | A wheel rolls along a zigzag curve as can be seen below. Which of the following pictures shows the curve that is described by the centre of the wheel?
<image1> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1405 | A circle with radius 1 rolls along a straight line from point $K$ to point $L$, as shown, with $K L=11 \pi$. In which position is the circle when it has arrived in $L$?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
141 | A monkey has torn off a piece of Captain Jack's map.
<image1>
What does the piece the monkey has torn off look like?
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | B | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1416 | In the three regular hexagons shown, $X, Y$ and $Z$ describe in this order the areas of the grey shaded parts. Which of the following statements is true?
<image1> | [
"$X=Y=Z$",
"$Y=Z \\neq X$",
"$Z=X \\neq Y$",
"$X=Y \\neq Z$",
"Each of the areas has a different value."
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
142 | These five animals are made up from different shapes. There is one shape which is only used on one animal. On which animal is this shape used?
<image1> | [
"A",
"B",
"C",
"D",
"E"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1426 | Five identical measuring jugs are filled with water. Four of them contain exactly the same amount of water. Which measuring jug contains a different amount?
<image1> | [
"A",
"B",
"C",
"D",
"E"
] | B | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1436 | When Cosme correctly wears his new shirt, as shown on the left figure, the horizontal stripes form seven closed arches around his body. This morning he buttoned his shirt in the wrong way, as shown on the right. How many open arches were there around Cosme's body this morning?
<image1> | [] | 1 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1438 | There are several figures that can be formed by nine squares of $1 \mathrm{~cm}$ side by side (see an example beside) and one of them has the biggest perimeter. What is this perimeter?
<image1> | [
"$12 \\mathrm{~cm}$",
"$14 \\mathrm{~cm}$",
"$16 \\mathrm{~cm}$",
"$18 \\mathrm{~cm}$",
"$20 \\mathrm{~cm}$"
] | E | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1452 | A park is shaped like an equilateral triangle. A cat wants to walk along one of the three indicated paths (thicker lines) from the upper corner to the lower right corner. The lengths of the paths are $P, Q$ and $R$, as shown. Which of the following statements about the lengths of the paths is true?
<image1> | [
"$P<Q<R$",
"$P<R<Q$",
"$P<Q=R$",
"$P=R<Q$",
"$P=Q=R$"
] | B | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1456 | Ally drew 3 triangles on a grid. Exactly 2 of them have the same area, exactly 2 of them are isosceles, and exactly 2 are right-angled triangles. 2 of the triangles are shown. Which could be the third one?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | D | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1466 | Various symbols are drawn on a piece of paper (see picture). The teacher folds the left side along the vertical line to the right. How many symbols of the left side are now congruent on top of a symbol on the right side?
<image1> | [] | 3 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1478 | One square is drawn inside each of the two congruent isosceles right-angled triangles. The area of square $P$ is 45 units. How many units is the area of square R?
<image1> | [] | 40 | null | Math | 2D Transformation | MathVision | Open-ended | |||
1483 | A dark disc with two holes is placed on the dial of a watch as shown in the diagram. The dark disc is now rotated so that the number 10 can be seen through one of the two holes. Which of the numbers could one see through the other hole now? <image1> | [
"2 and 6",
"3 and 7",
"3 and 6",
"1 and 9",
"2 and 7"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1500 | This piece of paper was folded in half twice, and then had two equilateral triangles cut out of it. Which diagram shows how the paper will look when it is unfolded again? <image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | C | The diagram shows how the cut-out triangles form rhombic holes when the paper is unfolded. 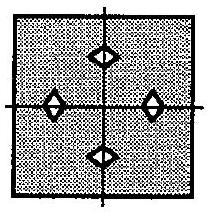 | Math | 2D Transformation | MathVision | Multiple Choice | |||
1504 | A transparent square sheet of film lies on a table. The letter $\mathbf{Y}$ is drawn (like this) on the sheet. We turn the sheet clockwise through $90^{\circ}$, then turn it over what is now the left edge of the sheet, and then turn it through $180^{\circ}$. Which figure can we now see?
<image1> | [
"A",
"B",
"C",
"D",
"E"
] | A | After turning clockwise through $90^{\circ}$, the letter will appear as <. Turning it over the left edge of the sheet gives $>$. Then a turn through $180^{\circ}$ gives $<$. | Math | 2D Transformation | MathVision | Multiple Choice | |||
1521 | Consecutive numbers have been entered diagonally criss-crossing the square on the right. Which of the following numbers could $x$ not be? <image1> | [
"128",
"256",
"81",
"121",
"400"
] | A | Since $x$ is the largest number entered, it is a square number and 128 is the only option which is not. | Math | 2D Transformation | MathVision | Multiple Choice | |||
1528 | The diagram shows a length of string wound over and under $n$ equal circles. The sum of the diameters of the circles is $d \mathrm{~cm}$. What is the length of the string in $\mathrm{cm}$ ?
<image1> | [
"$\\frac{1}{2} \\pi d$",
"$\\pi d n$",
"$2 \\pi d n$",
"$\\pi d$",
"$d n$"
] | A | Because the diameter of each circle is $\frac{d}{n} \mathrm{~cm}$, the length of each semicircular arc is $\frac{1}{2} \pi \frac{d}{n} \mathrm{~cm}$. For $n$ semicircles, this gives a total length of $\frac{1}{2} \pi \frac{d}{n} \times n \mathrm{~cm}=\frac{\pi}{2} d \mathrm{~cm}$. | Math | 2D Transformation | MathVision | Multiple Choice | |||
154 | Mr Beaver re-arranges the parts to build a kangaroo.
<image1>
Which part is missing?
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1540 | <image1>
In the diagram above there are 11 cards, each printed with two letters. The diagram below shows a rearangement of the cards, but only the top letters are shown.
<image2>
Which one of the following sequences of letters could appear on the bottom row of the second diagram? | [
"ANJAMKILIOR",
"RLIIMKOJNAA",
"JANAMKILIRO",
"RAONJMILIKA",
"ANMAIKOLIRJ"
] | E | The table below shows all the possible letters from KILIMANJARO underneath the rearranged letters of MISSISSIPPI: 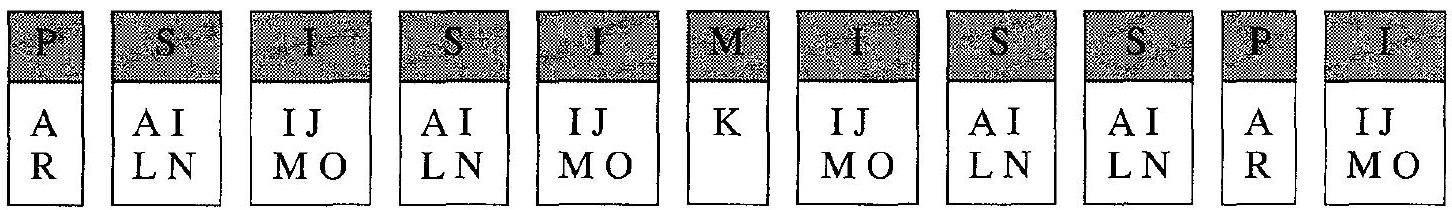 In options A, B, C and D the underlined letters are not possibl ANJAMKILIOR RLIMKOJNAA JANAMKILIRO RAONJMULIKA whereas option E, ANMAIKOLIRJ, is a possible rearrangement. | Math | 2D Transformation | MathVision | Multiple Choice | |||
1542 | What is the least possible number of small squares that we should shade in the diagram on the right for the whole diagram to have a line of symmetry? <image1> | [] | 3 | One can shade three squares to give a line of symmetry $L_{1}$ as shown on the right. This is the least, because $L_{2}, L_{3}$ or $L_{4}$ require an additional 4, 5 and 5 squares respectively to be shaded in order to be lines of symmetry. 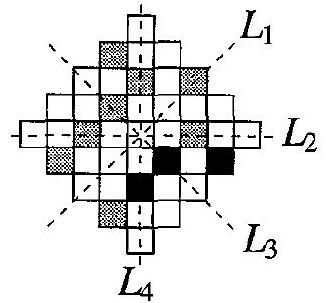 | Math | 2D Transformation | MathVision | Open-ended | |||
1550 | Marta wants to use 16 square tiles like the one shown to form a $4 \times 4$ square design. The tiles may be turned. Each arc bisects the sides it meets and has length $p \mathrm{~cm}$. She is trying to make the arcs connect to make a long path. What is the length, in centimetres, of the longest possible path? <image1> | [
"$15 p$",
"$20 p$",
"$21 p$",
"$22 p$",
"$25 p$"
] | D | The diagram on the right shows one way to join 22 arcs for a total length of $22 p \mathrm{~cm}$. This is the maximal length as, in order to use as many as possible of the 32 arcs, one cannot use 4 of the corners or more than 2 of the arcs in touching the outside of the square. 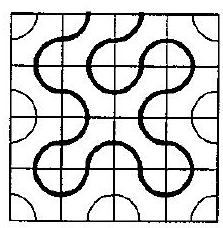 [Alternatively: Each edge tile has at least 1 arc meeting the edge. Thus there are at least 12 such arcs. Of these no more than 2 can belong to a path, because each is an end of that path. Thus $22 p$ is the maximum feasible length: and such a path is shown above.] | Math | 2D Transformation | MathVision | Multiple Choice | |||
1552 | How many different squares can be drawn in total by joining the dots with line segments in the part of the square lattice as shown on the right? <image1> | [] | 4 | There are four possible squares: 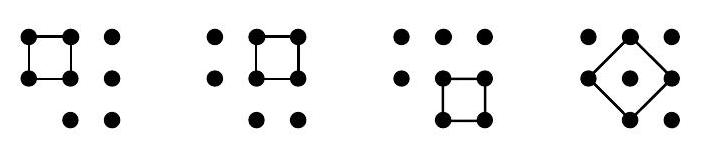 | Math | 2D Transformation | MathVision | Open-ended | |||
1571 | The diagram (which is not drawn to scale) shows a box measuring $5 \mathrm{~cm}$ by $5 \mathrm{~cm}$. There are seven bars in the box, each measuring $1 \mathrm{~cm}$ by $3 \mathrm{~cm}$. Kanga wants to slide the bars in the box so there is room for one more bar. What is the minimum number of bars that Kanga needs to move?
<image1> | [] | 3 | Label three of the bars $\mathrm{X}, \mathrm{Y}$ and $\mathrm{Z}$ as shown in the diagram. At the start Kanga can only move bar X down. Now Y and $\mathrm{Z}$ are the only bars that can be moved and Kanga must slide these bars to the left. Only now is there space for one more bar and Kanga has moved 3 bars. 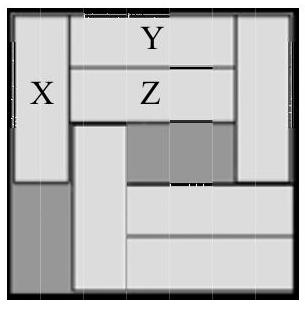 | Math | 2D Transformation | MathVision | Open-ended | |||
1581 | The diagram shows three squares. The medium square is formed by joining the midpoints of the sides of the large square. The small square is formed by joining the midpoints of the sides of the medium square. The area of the small square is $6 \mathrm{~cm}^{2}$. What is the difference between the area of the medium square and the area of the large square? <image1> | [
"$3 \\mathrm{~cm}^{2}$",
"$6 \\mathrm{~cm}^{2}$",
"$9 \\mathrm{~cm}^{2}$",
"$12 \\mathrm{~cm}^{2}$",
"$15 \\mathrm{~cm}^{2}$"
] | D | The diagram on the right shows how the shape can be dissected into sixteen congruent triangles. The small square has been dissected into four triangles, each of area $6 \div 4=1.5 \mathrm{~cm}^{2}$. The difference in area between the medium and the large square is eight of these triangles, that is $8 \times 1.5=12 \mathrm{~cm}^{2}$. 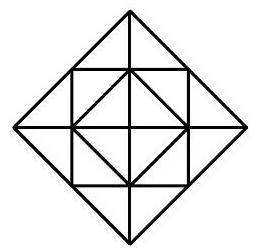 | Math | 2D Transformation | MathVision | Multiple Choice | |||
1584 | Lina has placed two shapes on a $5 \times 5$ board, as shown in the picture on the right. Which of the following five shapes should she place on the empty part of the board so that none of the remaining four shapes will fit in the empty space that is left? (The shapes may be rotated or turned over, but can only be placed so that they cover complete squares.)
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | D | By inspection, it is possible to spot the answer is shape $\mathrm{D}$. We can justify this as follows: When Lina places any of the other shapes on the empty part of the board, the shape must cover at least one square on the bottom row so that Lina cannot place shape $\mathrm{C}$ on the board.  There are five ways that Lina can place shape A on the board, covering at least one square on the bottom row, as shown below. In the first diagram, she can then place shape $\mathrm{E}$ on the board. In the second and third diagrams, she can then place shape $\mathrm{D}$ on the board. In the final two diagrams, she can then place shapes D or E on the board. 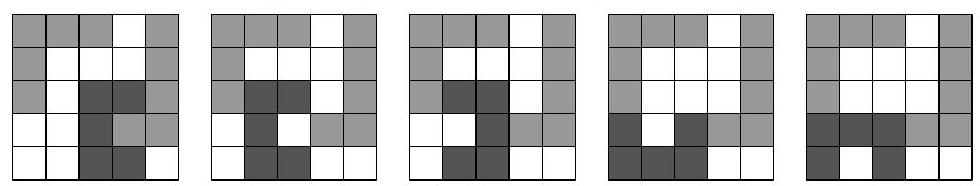 There is only one way that Lina can place shape B on the board, covering at least one square on the bottom row, as shown alongside. Lina can then place shapes D or E on the board.  There are four ways that Lina can place shape D on the board, covering at least one square on the bottom row, as shown below. In the first diagram, Lina can then place shape A on the board. In the second diagram, she can then place shape E on the board. In the third diagram, she can then place shape A on the board. In the fourth diagram, Lina cannot place any of the remaining shapes on the board. This is the shape she should choose.  Checking shape $\mathrm{E}$ shows that there are five ways it can be placed on the board, covering at least one square on the bottom row, as shown below. In the first two diagrams, Lina can then place shape A or D on the board. In the last three diagrams, she can then place shape $\mathrm{D}$ on the board.  | Math | 2D Transformation | MathVision | Multiple Choice | |||
1585 | The diagram on the right shows a square with side $3 \mathrm{~cm}$ inside a square with side $7 \mathrm{~cm}$ and another square with side $5 \mathrm{~cm}$ which intersects the first two squares. What is the difference between the area of the black region and the total area of the grey regions? <image1> | [
"$0 \\mathrm{~cm}^{2}$",
"$10 \\mathrm{~cm}^{2}$",
"$11 \\mathrm{~cm}^{2}$",
"$15 \\mathrm{~cm}^{2}$",
"more information needed"
] | D | Let the area of the white hexagon be $x \mathrm{~cm}^{2}$, as indicated in the diagram. Then the black area is $49-(9+x)=(40-x) \mathrm{cm}^{2}$. The total of the grey areas is $(25-x) \mathrm{cm}^{2}$. Thus the difference between the areas of the black and grey  regions is $(40-x)-(25-x)=15 \mathrm{~cm}^{2}$. | Math | 2D Transformation | MathVision | Multiple Choice | |||
1586 | The first diagram on the right shows a shape constructed from two rectangles. The lengths of two sides are marked: 11 and 13. The shape is cut into three parts and the parts are rearranged, as shown in the second diagram on the right. What is the length marked $x$ ?
<image1> | [] | 37 | The original shape constructed from two rectangles has base of length $11+13=24$. By considering the rearrangement, the lengths 11,13,24 and $x$ can be identified as shown in the diagram. Hence $x=13+24=37$.  | Math | 2D Transformation | MathVision | Open-ended | |||
1599 | Olivia and a friend are playing a game of 'battleships' on a $5 \times 5$ board. Olivia has already placed two ships as shown. She still has to place a $3 \times 1$ ship so that it covers exactly three cells. No two ships can have a boundary point in common. How many positions are there for her $3 \times 1$ ship? <image1> | [] | 8 | The $3 \times 1$ ship can be placed in two positions horizontally and six positions vertically as shown making a total of eight positions. 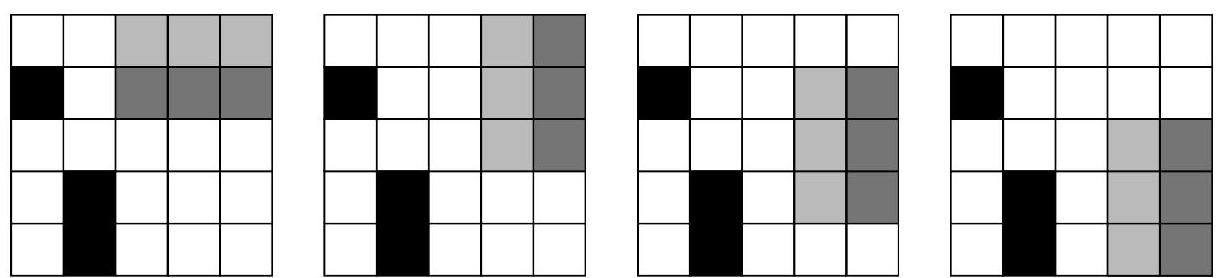 | Math | 2D Transformation | MathVision | Open-ended | |||
160 | Max wants to complete the jigsaw shown. He has different pieces.
<image1>
Which pieces does he have to use?
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice | |||
1609 | Rachel has several square pieces of paper of area $4 \mathrm{~cm}^{2}$. She cuts each of them into smaller squares and right-angled triangles in the manner shown in the first diagram. She takes some of the pieces and makes the shape shown in the second diagram.
What is the area in $\mathrm{cm}^{2}$ of the shape? <image1> | [] | 6 | The shape is made up of four pieces from one large square plus three further pieces making up half of a large square. Hence the area in $\mathrm{cm}^{2}$ is $4+\frac{1}{2} \times 4=6$. | Math | 2D Transformation | MathVision | Open-ended | |||
1616 | A $5 \times 5$ square is made from $1 \times 1$ tiles, all with the same pattern, as shown. Any two adjacent tiles have the same colour along the shared edge. The perimeter of the $5 \times 5$ square consists of black and white segments of length 1 . What is the smallest possible number of black segments on the perimeter of the
<image1>
$5 \times 5$ square? | [] | 5 | All the tiles on the perimeter of the $5 \times 5$ square contribute either one or two segments of length 1 to the perimeter with those contributing two segments being the four tiles at the corners. Each tile has only one white edge so there must be a minimum of four black segments on the perimeter. Now consider the central $3 \times 3$ square of the larger square. Adjacent tiles must be the same colour along a common edge so only eight of the nine tiles in the central square can have 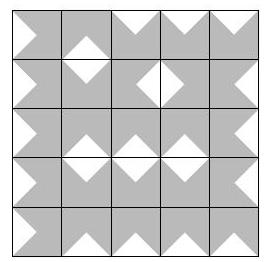 their white edge joined to another tile in the central square leaving at least one tile with its white edge not joined to any other tile in the central square. Hence, at least one tile on the perimeter must join its white edge to that of a tile from the central $3 \times 3$ square. The diagram above shows that an arrangement with only one tile on the perimeter joining its white edge to that of a central tile is possible. Hence, the smallest possible number of black segments on the perimeter of the $5 \times 5$ square is 5 . | Math | 2D Transformation | MathVision | Open-ended | |||
1632 | A $3 \mathrm{~cm}$ wide strip is grey on one side and white on the other. Maria folds the strip, so that it fits inside a rectangle of length $27 \mathrm{~cm}$, as shown. The grey trapeziums are identical. What is the length of the original strip?
<image1> | [
"$36 \\mathrm{~cm}$",
"$48 \\mathrm{~cm}$",
"$54 \\mathrm{~cm}$",
"$57 \\mathrm{~cm}$",
"$81 \\mathrm{~cm}$"
] | D | Let the length of the shorter of the two parallel sides of the grey trapeziums be $x$ $\mathrm{cm}$. Since the folded shape is $27 \mathrm{~cm}$ long and the strip is $3 \mathrm{~cm}$ wide, we have $3+x+3+x+3+x+3+x+3=27$ which has solution $x=3$. Hence the length of the longer of the two parallel sides of the grey trapezium is $(3+x+3) \mathrm{cm}=9 \mathrm{~cm}$. Also, since the height of each trapezium is equal to the width of the strip, the height is $3 \mathrm{~cm}$ and hence the height of each of the small rectangles is $(6-3) \mathrm{cm}$. Therefore the total length of the strip (along the edge marked in the diagram) is $$ (6+9+3+3+3+3+3+9+3+3+3+3+6) \mathrm{cm}=57 \mathrm{~cm} . $$ 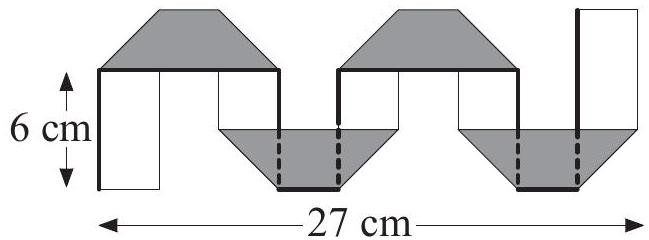 | Math | 2D Transformation | MathVision | Multiple Choice | |||
1660 | Dominoes are said to be arranged correctly if, for each pair of adjacent dominoes, the numbers of spots on the adjacent ends are equal. Paul laid six dominoes in a line as shown in the diagram.
<image1>
He can make a move either by swapping the position of any two dominoes (without rotating either domino) or by rotating one domino. What is the smallest number of moves he needs to make to arrange all the dominoes correctly? | [] | 3 | The dominoes in the line contain three ends with four spots and three ends with six spots, as shown in diagram 1. Therefore, a correctly arranged set of these dominoes will have four spots at one end and six spots at the other, as is currently the case. Hence, Paul does not need to move either of the end dominoes. If he swaps the third and the fifth dominoes from diagram 1, he obtains the row shown in diagram 2 which has the same number of spots in the adjacent ends of the fourth, fifth and sixth dominoes. Next, if he swaps the second and third dominoes from diagram 2 to obtain the line shown in diagram 3 , he has matched the spots at the adjacent ends of the first and second dominoes. Finally, rotating the third domino in diagram 3, he obtains the correctly arranged line as shown in diagram 4 . This shows that it is possible to arrange the dominos correctly in three moves. To see that two moves is not sufficient, note that, whatever else needs to happen, the two $1 \mathrm{~s}$ must be correctly placed next to each other. To do that requires one of the dominos with a 1 to be rotated and then one pair of dominos to be swapped so that the two $1 \mathrm{~s}$ are now next to each other. A similar argument applies to the two dominos with a 3. However, this is not possible in only two moves. Therefore the smallest number of moves he needs to make is 3 .  | Math | 2D Transformation | MathVision | Open-ended | |||
1667 | Which of the following $4 \times 4$ tiles cannot be formed by combining the two given pieces?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | E | When the two given pieces are joined together, any resulting square must have on its outside one row and one column, each of which have alternating black and white squares. Therefore tile E cannot be made. The diagrams below show how the tiles in options A, B, C and D can be made by combining the given pieces, confirming $\mathrm{E}$ as the only tile which cannot be made. 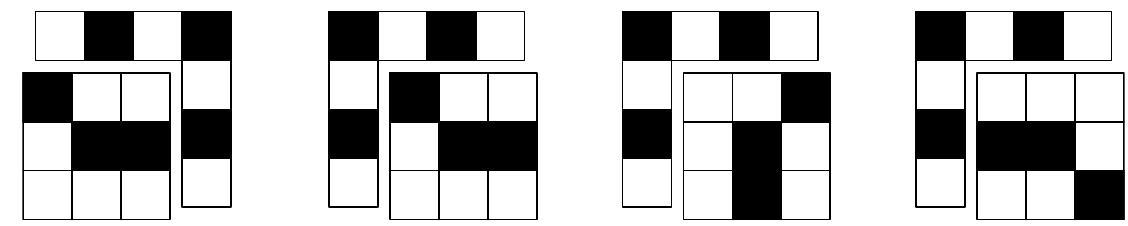 | Math | 2D Transformation | MathVision | Multiple Choice | |||
1675 | The diagram shows a shape made up of 36 identical small equilateral triangles. What is the smallest number of small triangles identical to these that could be added to the shape to turn it into a hexagon? <image1> | [] | 18 | To turn the figure given in the question into a hexagon by adding the smallest number of triangles, two triangles should be added to create each vertex of the hexagon (shaded dark grey) and one triangle added to create each edge  (shaded light grey) as shown on the right for one edge. Since a hexagon has six vertices and six edges, the smallest number of triangles required is $6 \times(2+1)=18$. | Math | 2D Transformation | MathVision | Open-ended | |||
1680 | Aisha has a strip of paper with the numbers $1,2,3,4$ and 5 written in five cells as shown. She folds the strip so that the cells overlap, forming 5 layers. Which of the following configurations, from top layer to bottom layer, is it not possible to obtain? <image1> | [
"$3,5,4,2,1$",
"$3,4,5,1,2$",
"$3,2,1,4,5$",
"$3,1,2,4,5$",
"$3,4,2,1,5$"
] | E | The four figures (A) to (D) give a side-view of how the strip could be folded to give the arrangements of numbers in options A to D. Figure (E) shows that it is not possible to get option E since number 5 would end up between number 4 and number 2 (as indicated by the dashed line labelled 5) rather than below number 1 as is required.   | Math | 2D Transformation | MathVision | Multiple Choice | |||
1681 | Bella took a square piece of paper and folded two of its sides to lie along the diagonal, as shown, to obtain a quadrilateral. What is the largest size of an angle in that quadrilateral? <image1> | [
"$112.5^{\\circ}$",
"$120^{\\circ}$",
"$125^{\\circ}$",
"$135^{\\circ}$",
"$150^{\\circ}$"
] | A | Since the quadrilateral is formed by folding the $45^{\circ}$ angles above and below the diagonal of the square in half, the size of the small angle of the quadrilateral is $2 \times\left(\frac{1}{2} \times 45^{\circ}\right)=45^{\circ}$. One angle of the quadrilateral is $90^{\circ}$ and the other two are equal from the construction. Therefore, since the sum of the angles in a quadrilateral is $360^{\circ}$, the size of the equal angles is $(360-90-45)^{\circ} \div 2=225^{\circ} \div 2=112.5^{\circ}$. | Math | 2D Transformation | MathVision | Multiple Choice | |||
1685 | When the five pieces shown are fitted together correctly, the result is a rectangle with a calculation written on it. What is the answer to this calculation? <image1> | [] | -100 | When you look at the pieces, you can see that the calculation both starts and ends with a piece with a "2" written on. Further, the only piece that can be placed next to the first " 2 " is the subtraction sign. Then the " 0 " must be attached to the final " 2 " and so the rectangle must be as shown. $$ 2 \nmid-q 1 q 0 \nmid 2 $$ Hence the answer to the calculation is -100 . | Math | 2D Transformation | MathVision | Open-ended | |||
1689 | A rectangular strip of paper of dimensions $4 \times 13$ is folded as shown in the diagram. Two rectangle s are formed with areas $P$ and $Q$ where $P=2 Q$. What is the value of $x$ ? <image1> | [] | 6 | Let the height of the rectangle $Q$ be $y$. Since the original $4 \times 13$ rectangle has been folded to form the second shape, both the width of the rectangle with area $Q$ and the height of the rectangle with area $P$ are 4 . Considering the base of the rectangle before and after folding gives the equation $x+4+y=13$ and hence $x+y=9$. Since the two rectangles both have one side of length 4, the condition $P=2 Q$ implies that $x=2 y$ and so $y=3$ and $x=6$. | Math | 2D Transformation | MathVision | Open-ended | |||
1708 | Kristina has a piece of transparent paper with some lines marked on it. She folds it along the central dashed line, as indicated. What can she now see? <image1> | [
"$2: 6: 9$",
"$2: 6: 6$",
"$5: 6: 9$",
"$2: 8: 6$",
"$5: 8: 9$"
] | C | Each digit in the completed number contains three horizontal line segments. Since the markings above the fold will appear the other way up when folded, the first digit in the completed number contains a vertical line top left and a vertical line bottom right in addition to the three horizontal lines and so is a 5. The second digit in the completed number contains a double length vertical line on the left and a vertical line bottom right in addition to the three horizontal lines and so is a 6. Similarly, the third digit in the completed number contains a double length vertical line on the right and a vertical line top left in addition to the three horizontal lines and so is a 9 . Therefore the number that will be seen is 569 . | Math | 2D Transformation | MathVision | Multiple Choice | |||
1744 | Henna has four hair ribbons of width $10 \mathrm{~cm}$. When she measures them, she finds that each ribbon is $25 \mathrm{~cm}$ longer than the next smallest ribbon. She then arranges the ribbons to form two different shapes as shown in the diagram. How much longer is the perimeter of the second shape than the perimeter of the first shape? <image1> | [
"$75 \\mathrm{~cm}$",
"$50 \\mathrm{~cm}$",
"$25 \\mathrm{~cm}$",
"$20 \\mathrm{~cm}$",
"$0 \\mathrm{~cm}$"
] | B | Let the length of the shortest ribbon be $x \mathrm{~cm}$. Therefore the lengths of the other ribbons are $(x+25) \mathrm{cm},(x+50) \mathrm{cm}$ and $(x+75) \mathrm{cm}$. The perimeter of the first shape (starting from the lower left corner and working clockwise) is $(x+10+25+10+25+10+25+10+x+75+40) \mathrm{cm}=(2 x+230) \mathrm{cm}$ while the perimeter of the second shape (again starting from the lower left corner) is $(x+50+10+25+10+50+10+75+10+x+40) \mathrm{cm}=(2 x+280) \mathrm{cm}$. Hence the difference between the two perimeters is $(2 x+280) \mathrm{cm}-(2 x+230) \mathrm{cm}=50 \mathrm{~cm}$. | Math | 2D Transformation | MathVision | Multiple Choice | |||
1748 | Three congruent isosceles trapeziums are assembled to form an equilateral triangle with a hole in the middle, as shown in the diagram.
<image1>
What is the perimeter of the hole? | [
"$3 a+6 b$",
"$3 b-6 a$",
"$6 b-3 a$",
"$6 a+3 b$",
"$6 a-3 b$"
] | E | Since the triangle formed when the trapeziums are put together is equilateral, the smaller angles in the isosceles trapeziums are both $60^{\circ}$. Consider one trapezium split into a parallelogram and a triangle as shown. 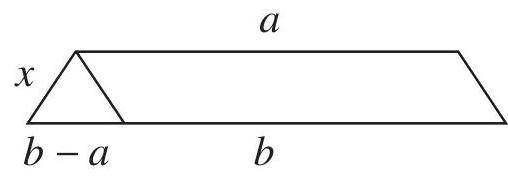 Since the original trapezium contains two base angles of $60^{\circ}$, the triangle also contains two base angles of $60^{\circ}$. Hence the triangle is equilateral and has side length $(b-a)$. Now consider the large equilateral triangle with the hole. The perimeter of the hole is $3(a-x)$ where $x$ is the length of the shortest sides of the trapezium. Therefore the perimeter of the hole is $3(a-(b-a))=3(2 a-b)=6 a-3 b$. | Math | 2D Transformation | MathVision | Multiple Choice | |||
1779 | Using only pieces like the one shown in the diagram, Zara wants to make a complete square without gaps or overlaps.
<image1>
What is the smallest number of pieces she can use? | [] | 20 | Since the total area of a square created with $N$ of the pieces shown will be $5 N$, we need $5 N$ to be a square number. Therefore $N$ is of the form $5 m^{2}$ for some integer $m$ and, of the options given, only 5 and 20 are of that form. Consider first whether it is possible to create a square with 5 pieces. Such a square would be a $5 \times 5$ square and, as shown in the diagrams below, it is then only possible to cover the central cell in one of two ways (rotations and reflections of the arrangements shown being essentially the same). 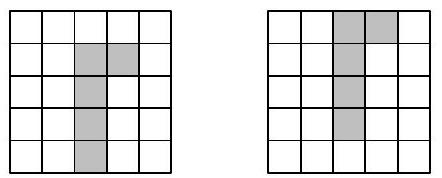 Whichever of these two arrangements is used to cover the central cell, it is easy to see that the remaining cells cannot be covered with four more of the pieces and hence it is impossible to build a square with 5 of the pieces shown. However, since two of the pieces can be placed next to each other to form a $5 \times 2$ rectangle as shown, 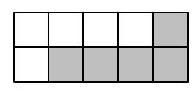 and 10 of these rectangles can easily be combined to create a $10 \times 10$ square, a square can be built using 20 pieces. | Math | 2D Transformation | MathVision | Open-ended | |||
1782 | Both of the shapes shown in the diagram are formed from the same five pieces, a $5 \mathrm{~cm}$ by $10 \mathrm{~cm}$ rectangle, two large quarter circles and two small quarter circles. What is the difference in $\mathrm{cm}$ between their perimeters? <image1> | [] | 20 | Both shapes have two large quarter circles and two small quarter circles in their perimeters. Additionally, the first shape has two edges of length $10 \mathrm{~cm}$ and two edges of length $5 \mathrm{~cm}$ in its perimeter, whereas the second shape has only one edge of length $10 \mathrm{~cm}$ in its perimeter. Therefore the difference, in $\mathrm{cm}$, between their perimeters is $10+5+5=20$. | Math | 2D Transformation | MathVision | Open-ended | |||
1789 | A square piece of paper of area $64 \mathrm{~cm}^{2}$ is folded twice, as shown in the diagram. What is the sum of the areas of the two shaded rectangles?
<image1> | [
"$10 \\mathrm{~cm}^{2}$",
"$14 \\mathrm{~cm}^{2}$",
"$15 \\mathrm{~cm}^{2}$",
"$16 \\mathrm{~cm}^{2}$",
"$24 \\mathrm{~cm}^{2}$"
] | D | Consider the square divided up into 16 congruent smaller squares, as shown. Since the original square has area $64 \mathrm{~cm}^{2}$, each of the smaller squares has area $4 \mathrm{~cm}^{2}$. When the square is folded twice in the manner shown, it can be seen that each of the shaded rectangles is made up of two small squares. 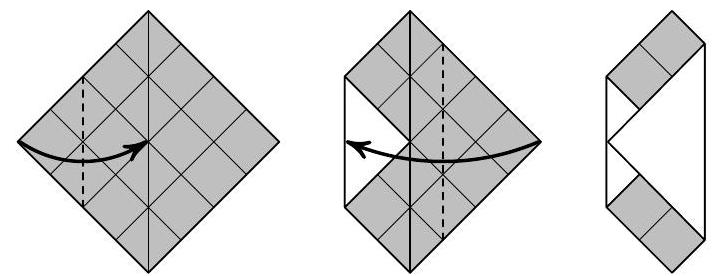 Therefore the sum of the areas of the two shaded rectangles is $2 \times 2 \times 4 \mathrm{~cm}^{2}=16 \mathrm{~cm}^{2}$. | Math | 2D Transformation | MathVision | Multiple Choice | |||
179 | A square piece of paper has been cut in three pieces. Two of them are in the picture on the right. What is the third one?
<image1>
<image2> | [
"A",
"B",
"C",
"D",
"E"
] | A | null | Math | 2D Transformation | MathVision | Multiple Choice |